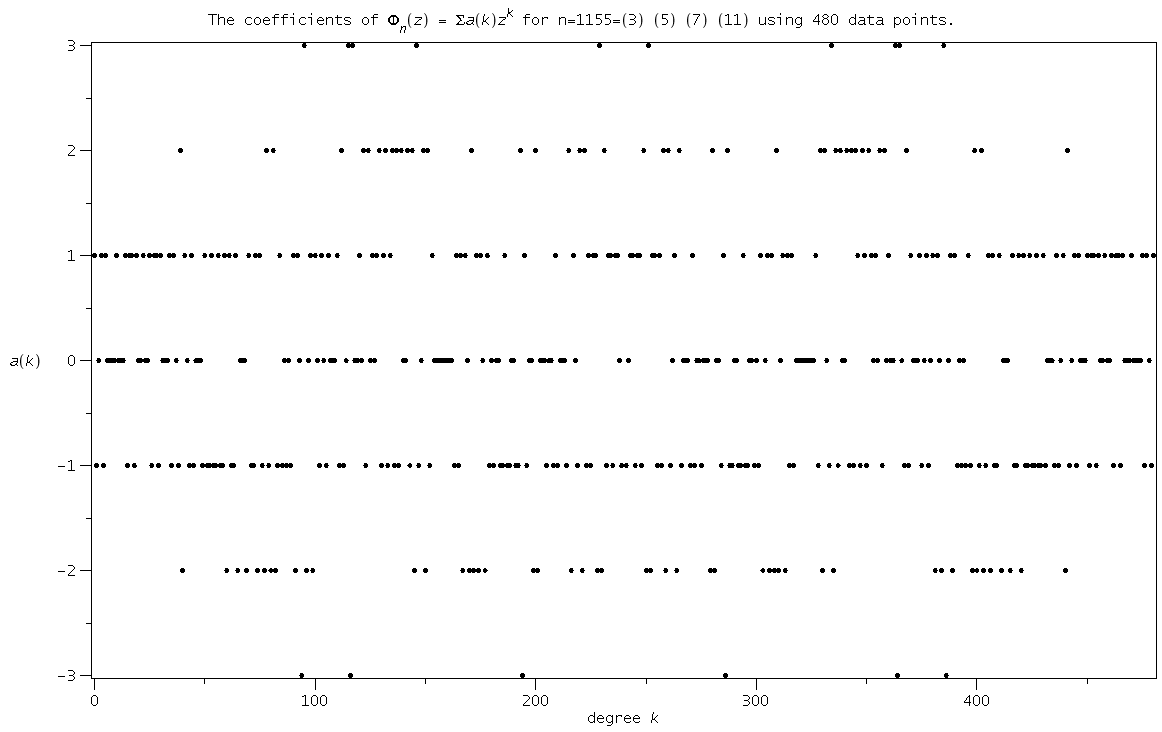
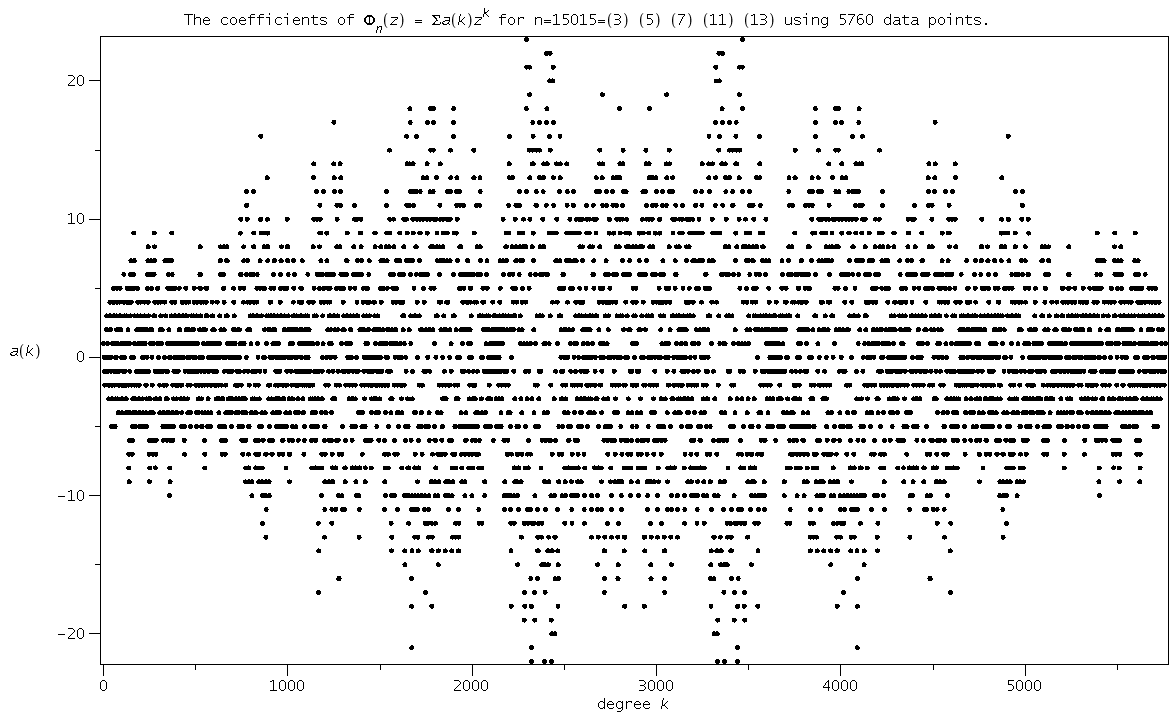
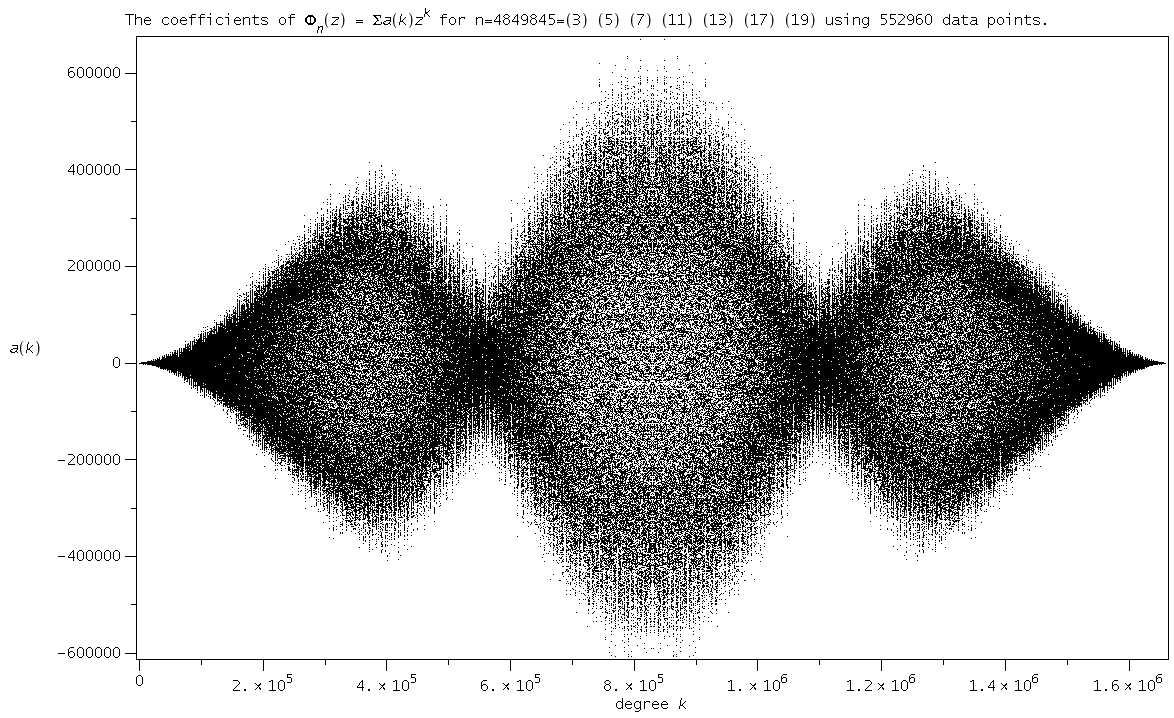
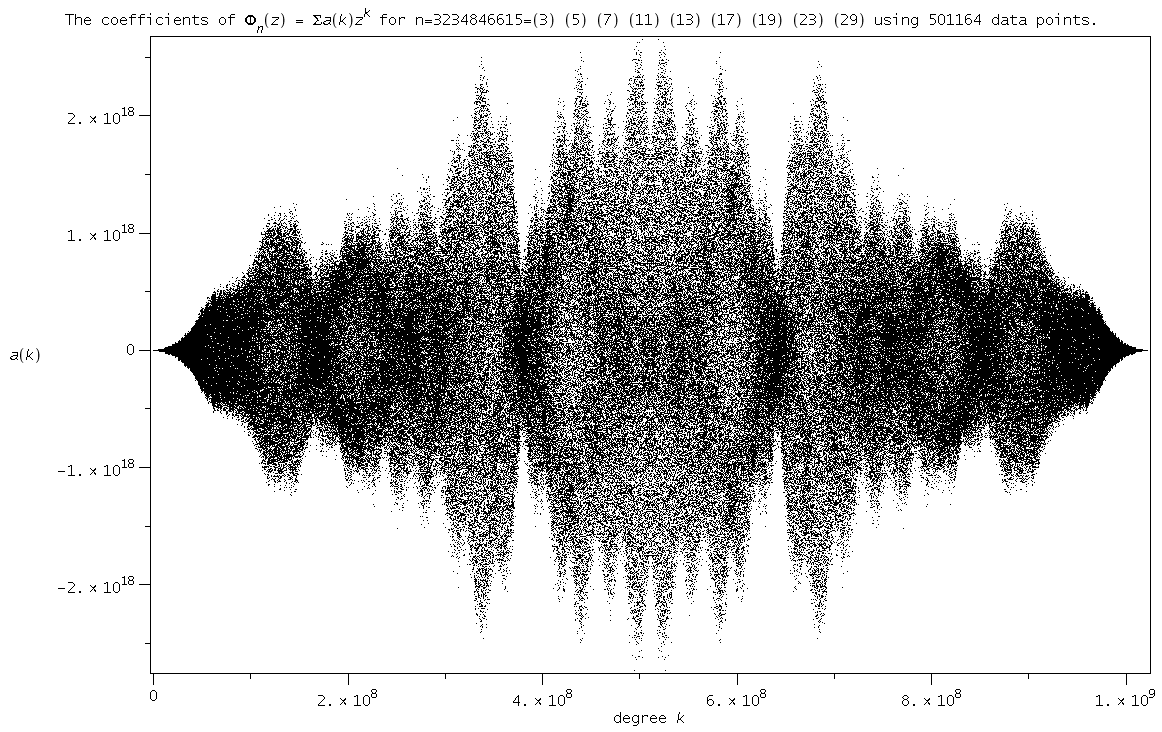
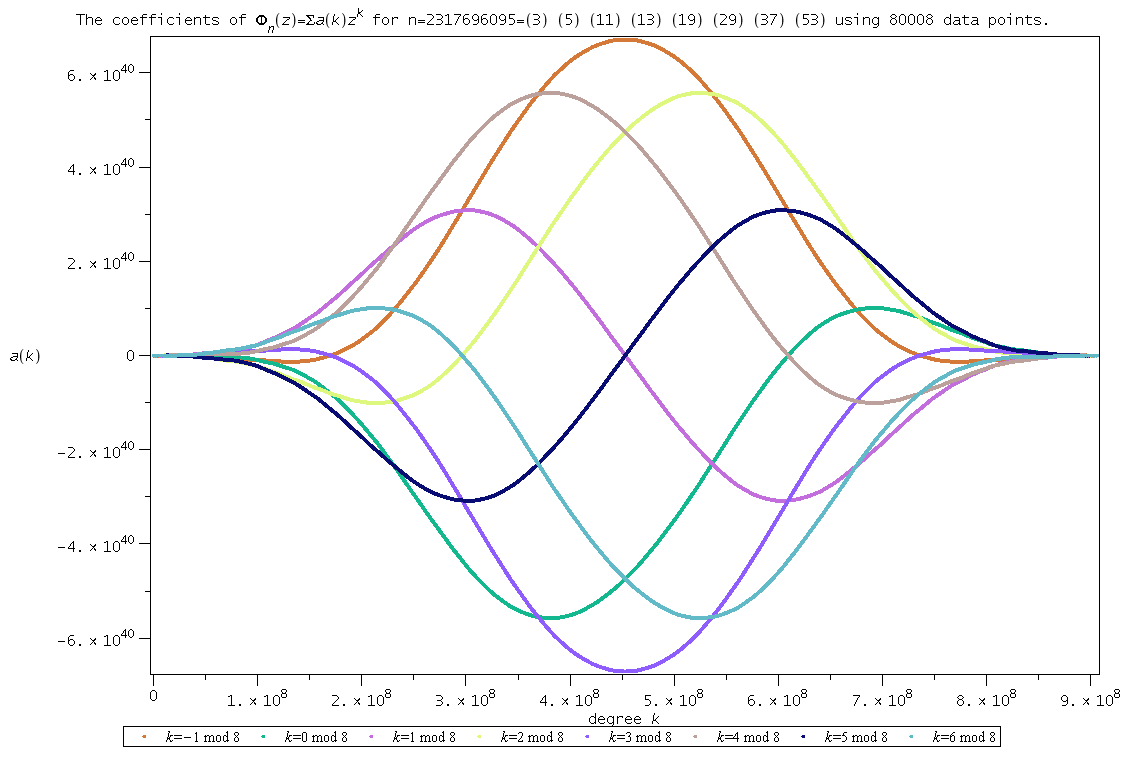
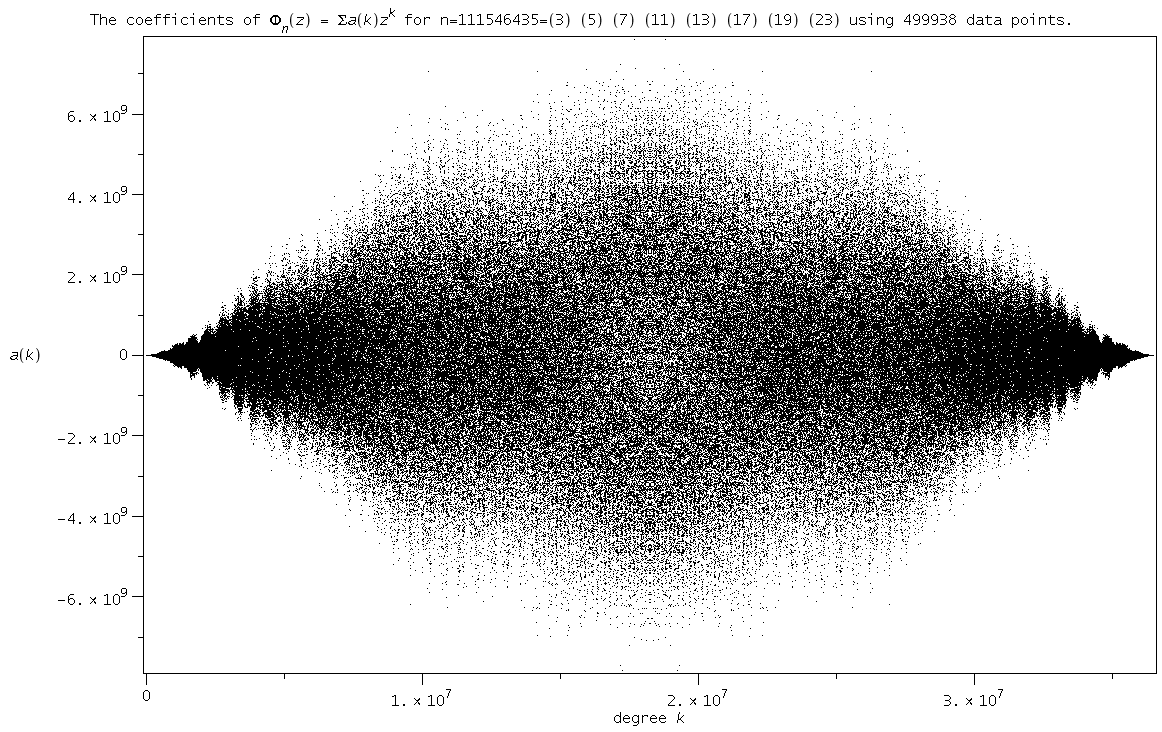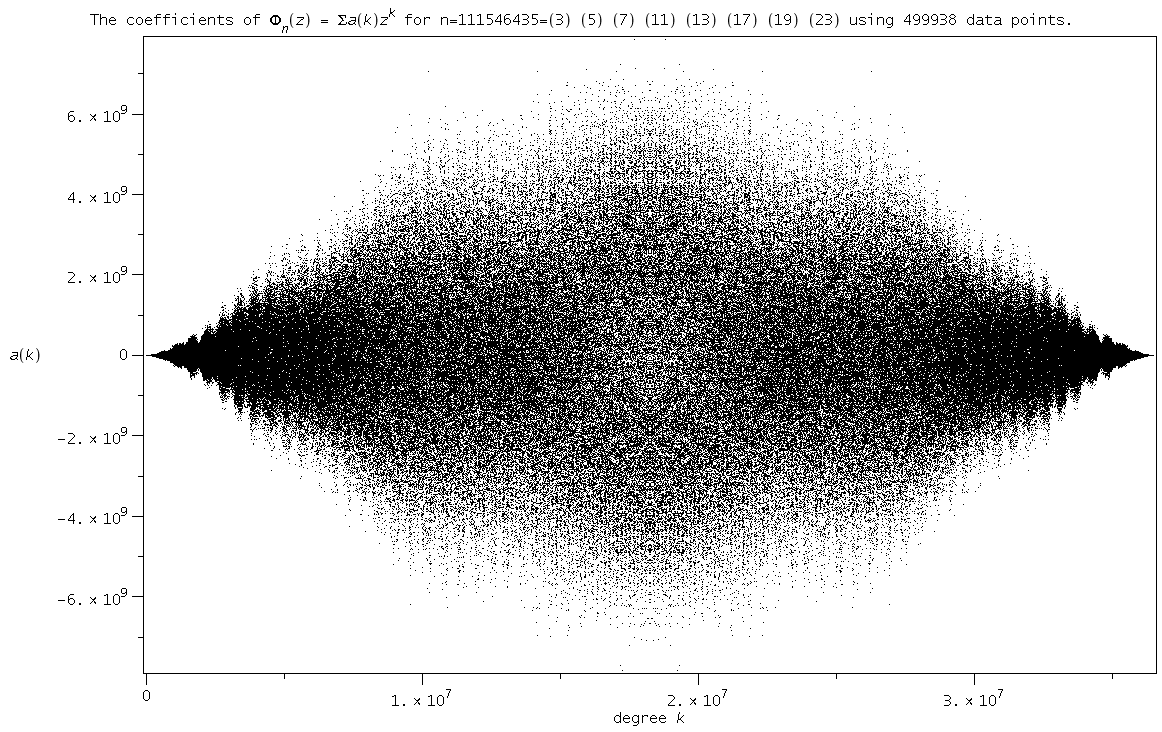We include here some plots of cyclotomic polynomials, namely some of particularly large height. We generate a random subset (of manageable size) of terms of $\Phi_n(z)$ and plot those using Maple. We mention in each plot how many points we plotted. We also include the factorization of $n$. It is interesting that the plots of coefficients of $\Phi_{np}(z)$ oftencase looks similar to that of $\Phi_n(z)$. You'll observe the terms form bands in our plots. In a plot with $k$ bands, it appears that each of the bands is comprised of terms whose degree belongs to a particular congruence class modulo $k$. It suggest to us that $(z^k-1)\Phi_n(z)$ has an appreciably smaller height than that of $\Phi_n(z)$.

---

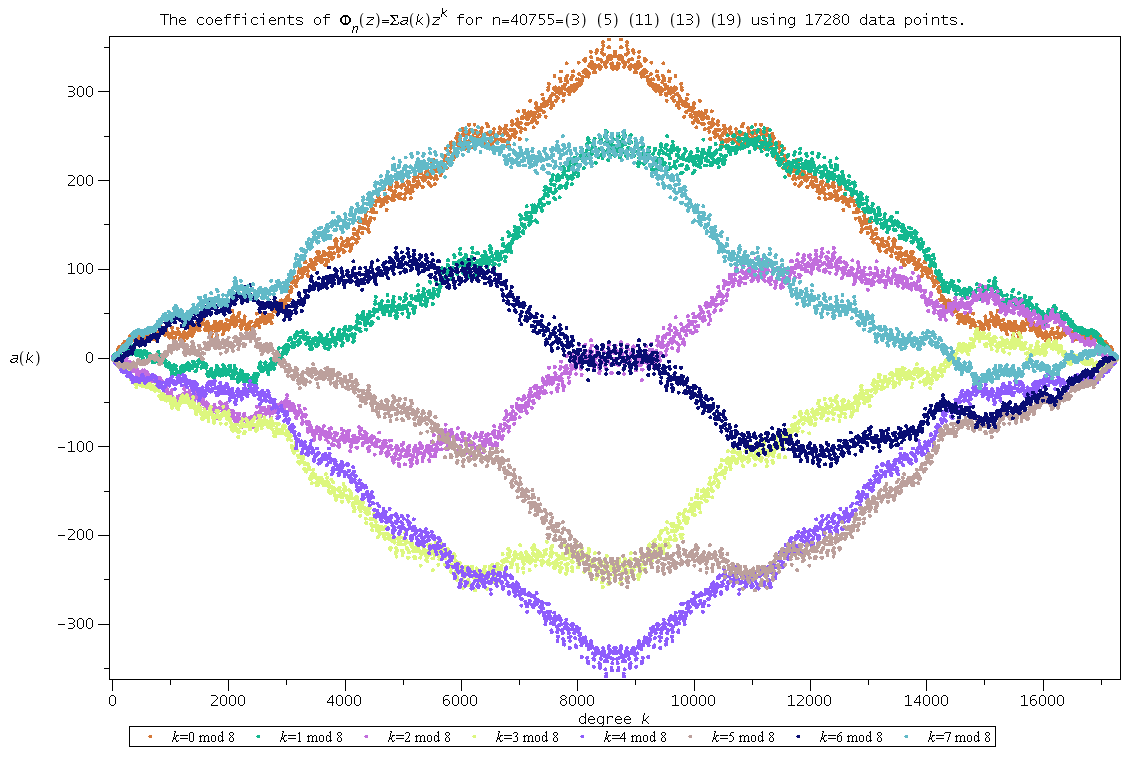
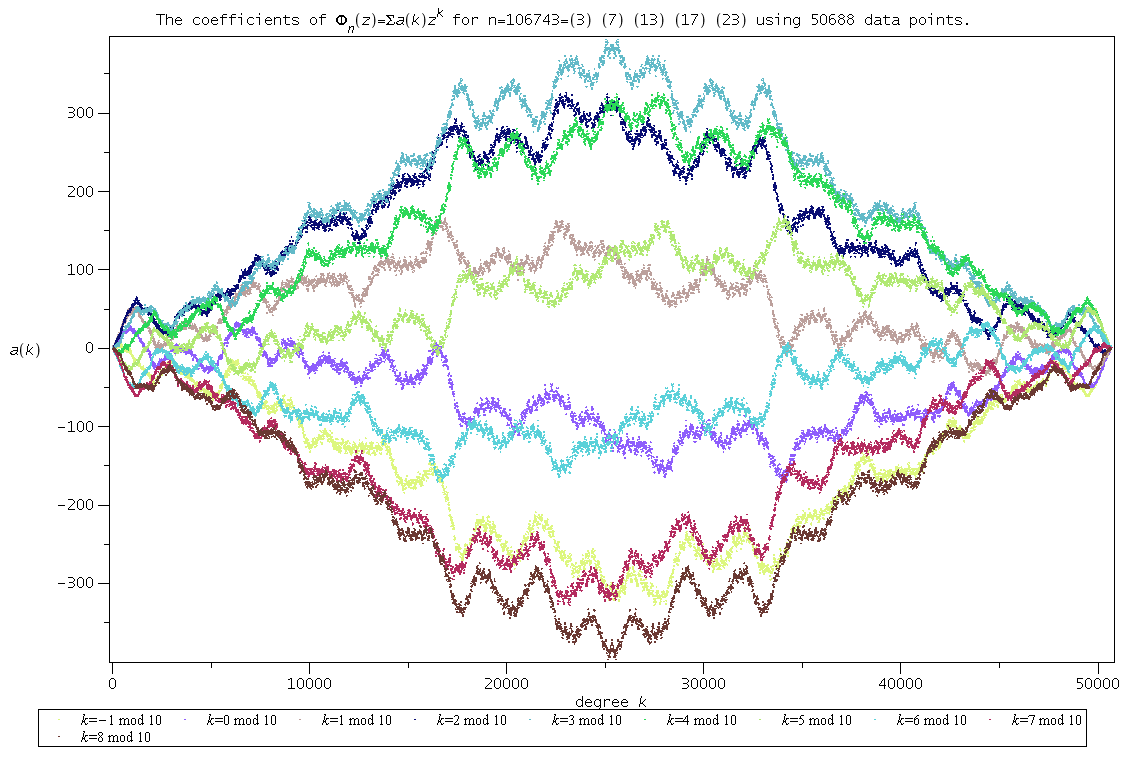
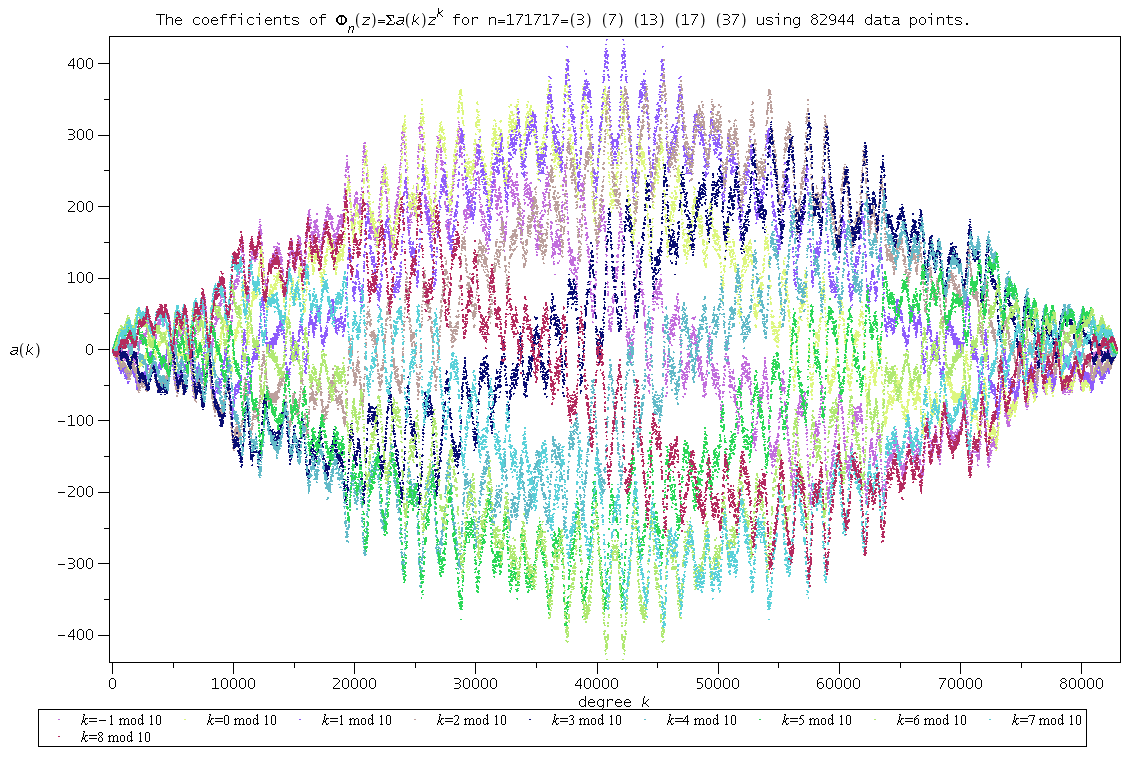
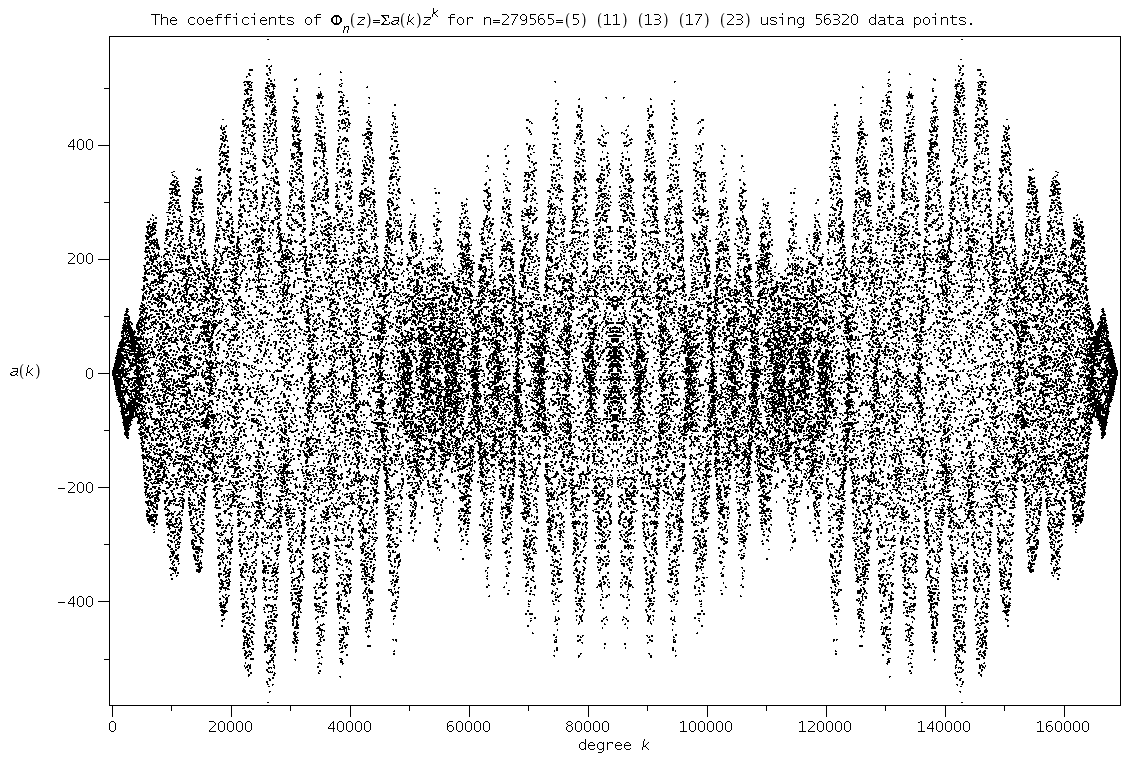
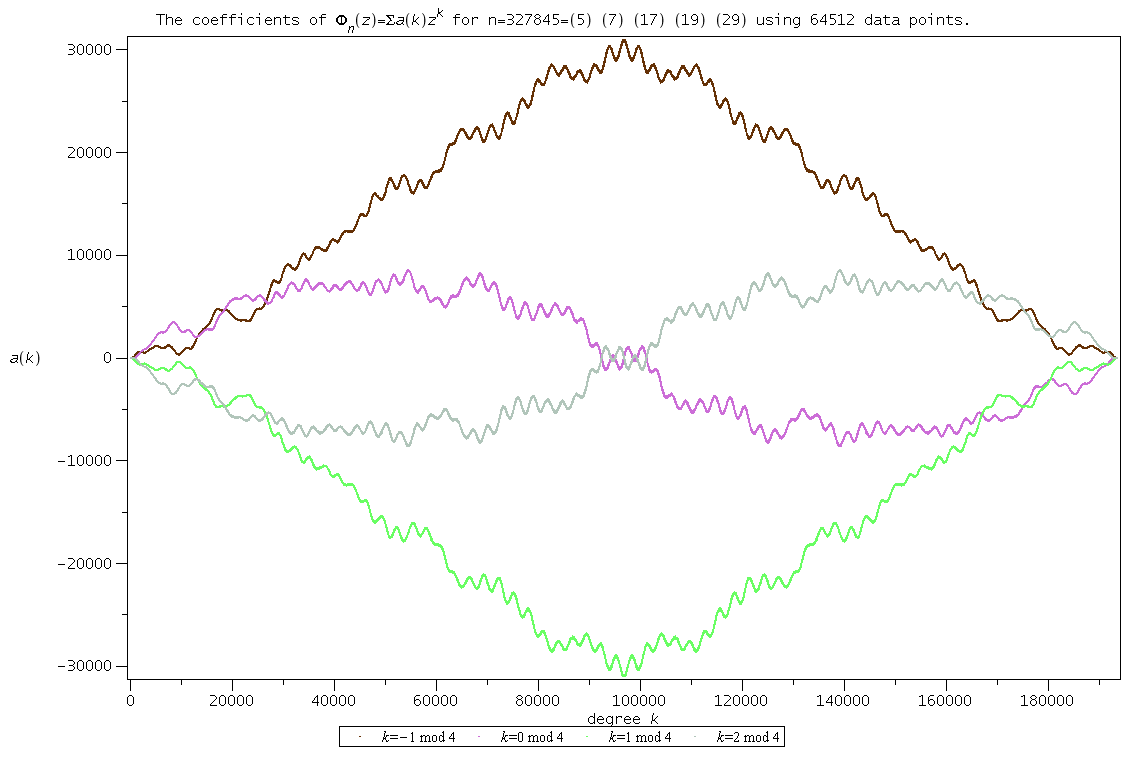
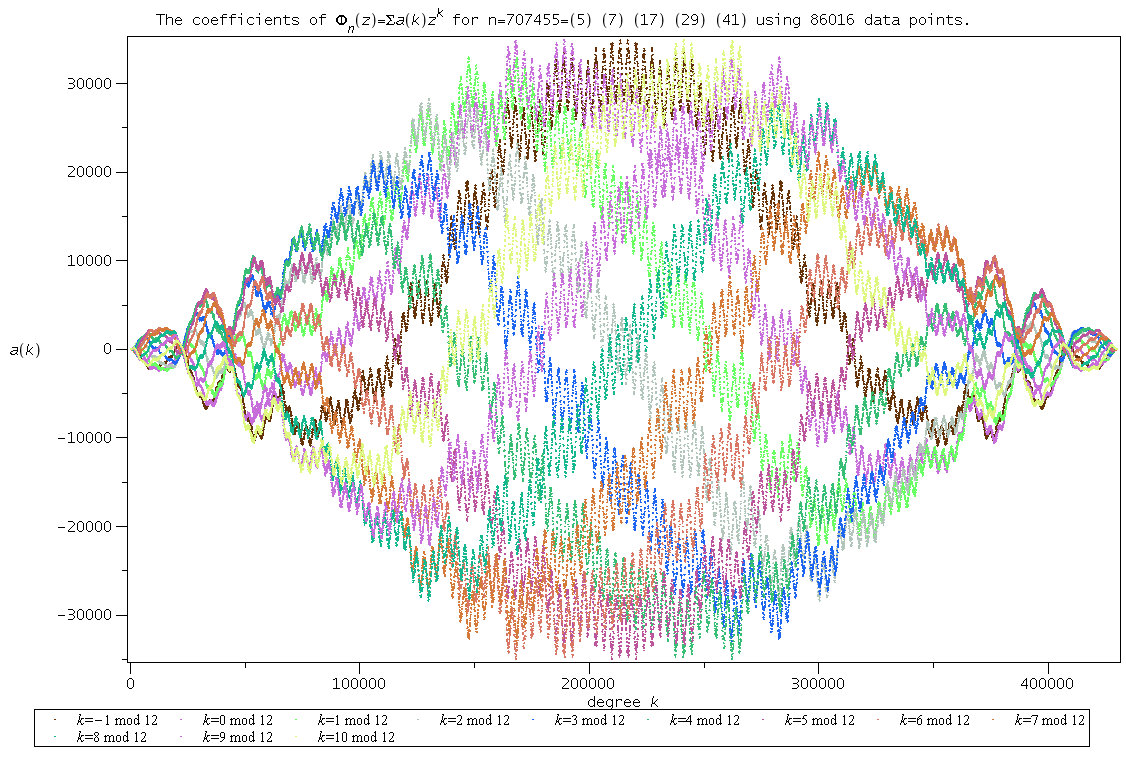
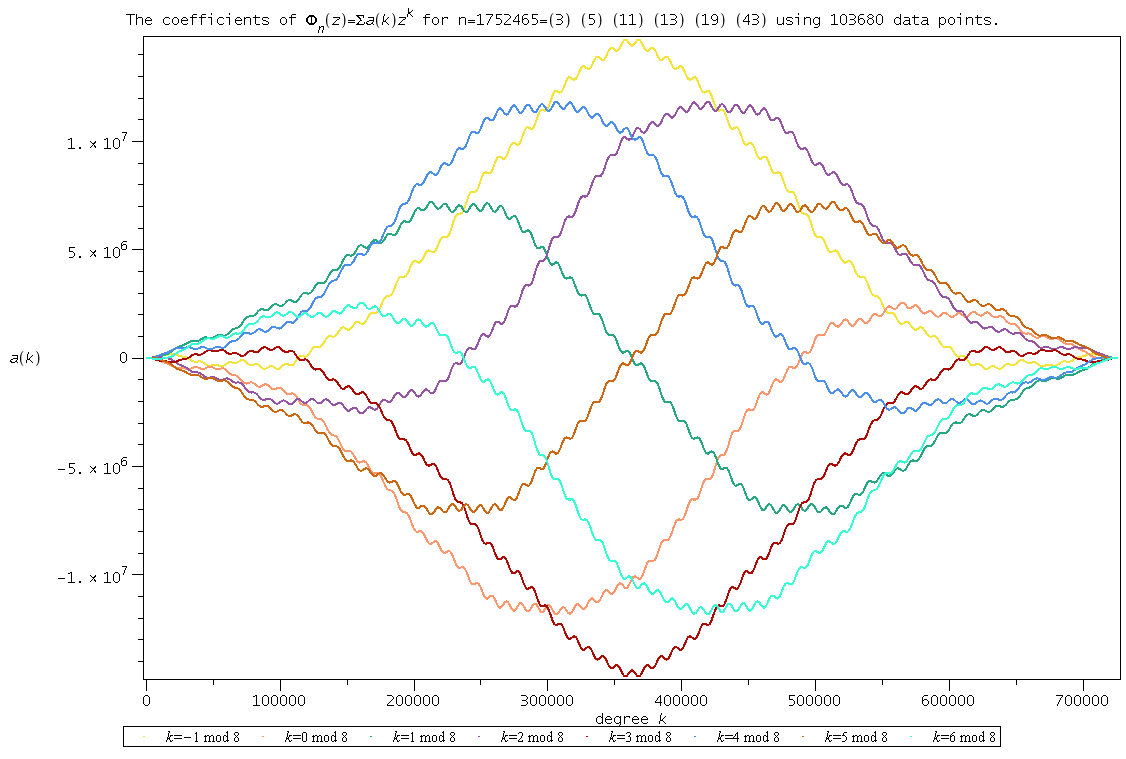
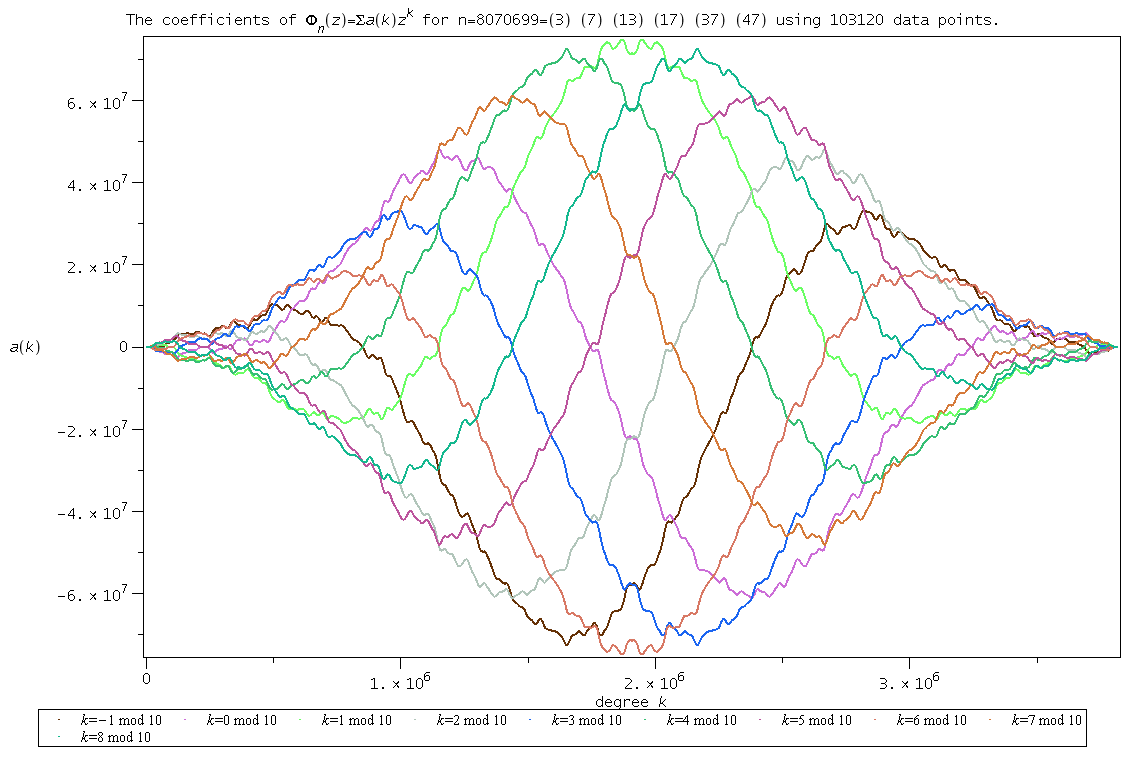
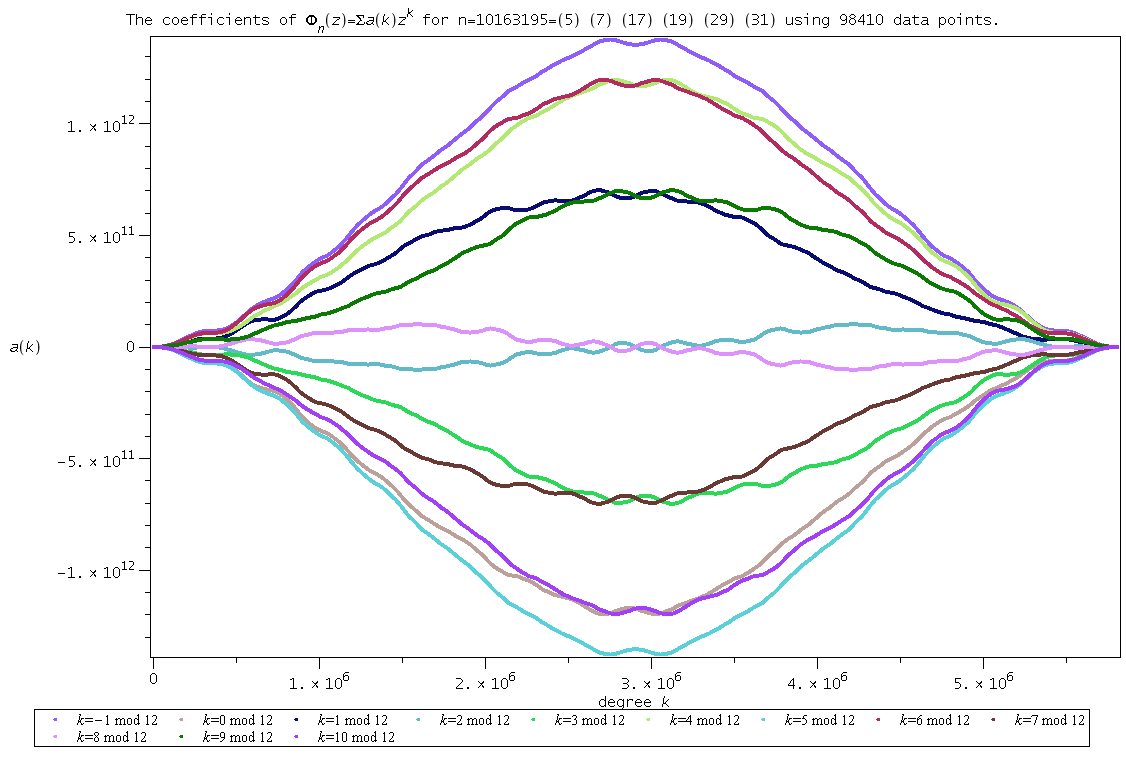
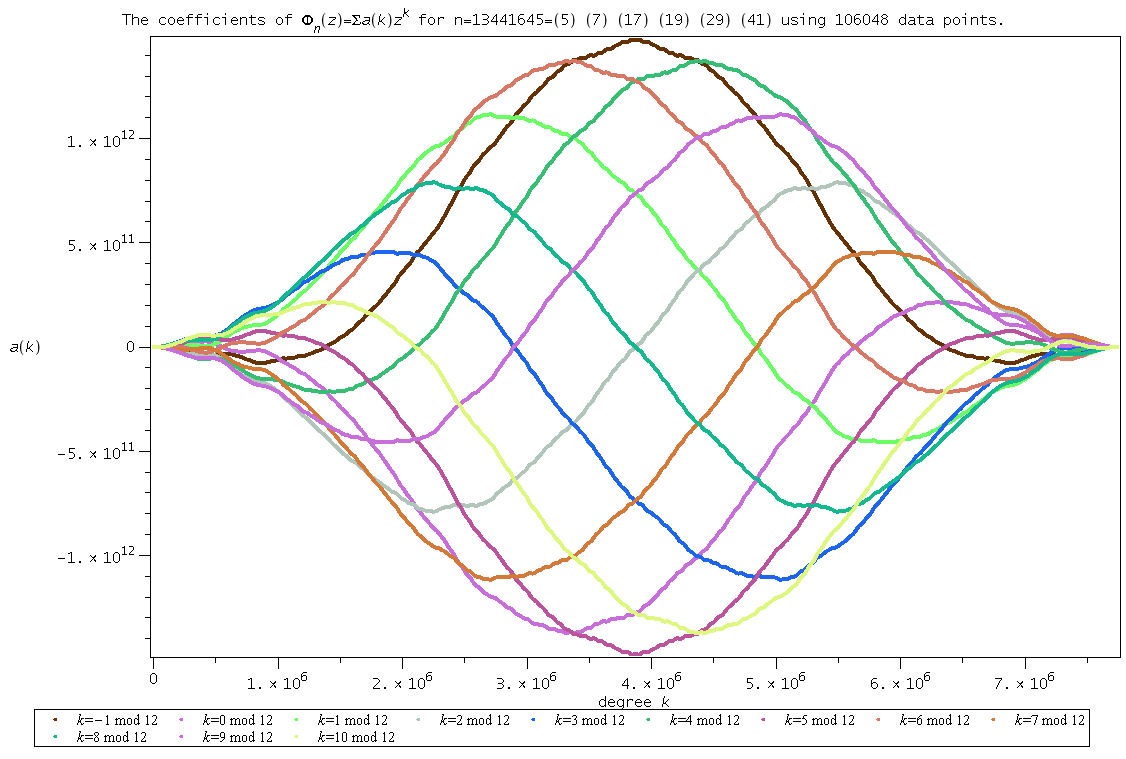
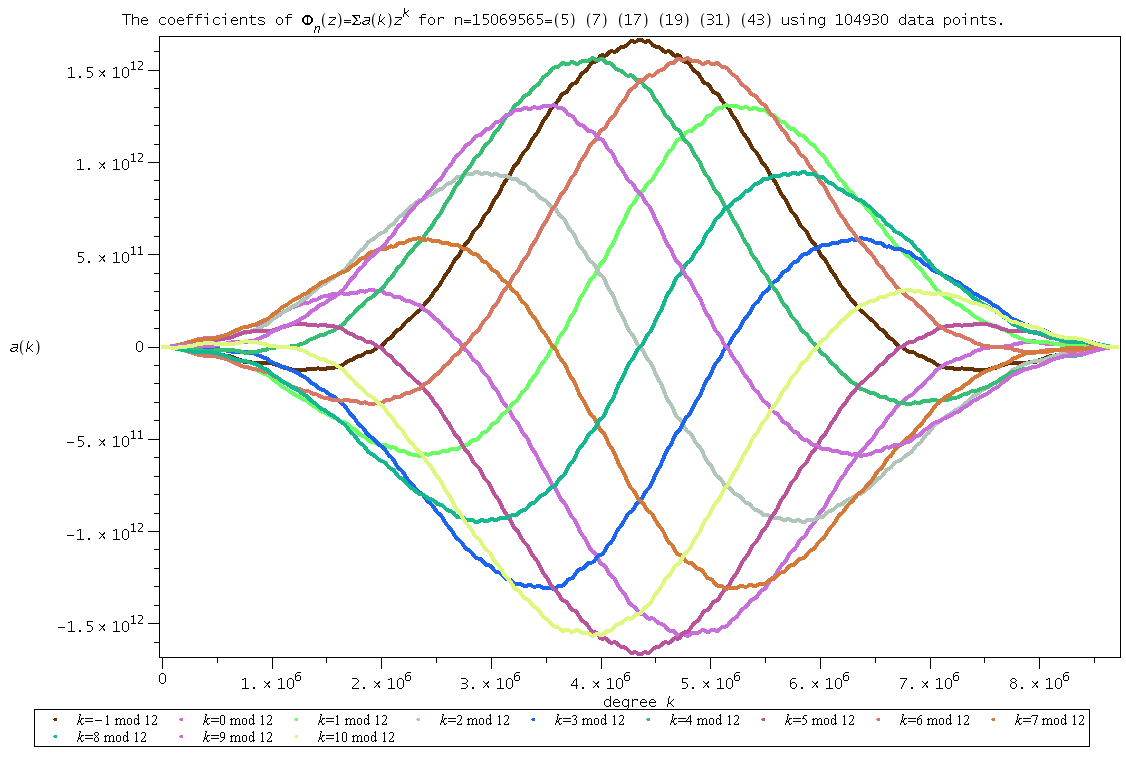
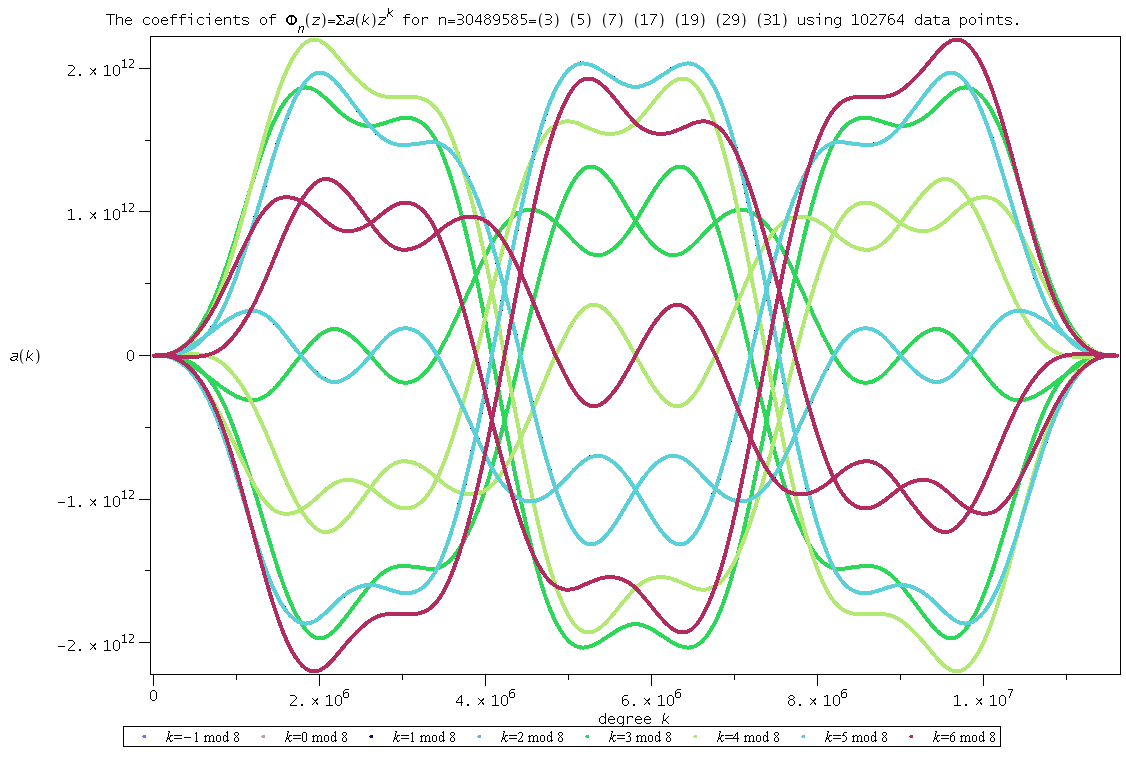
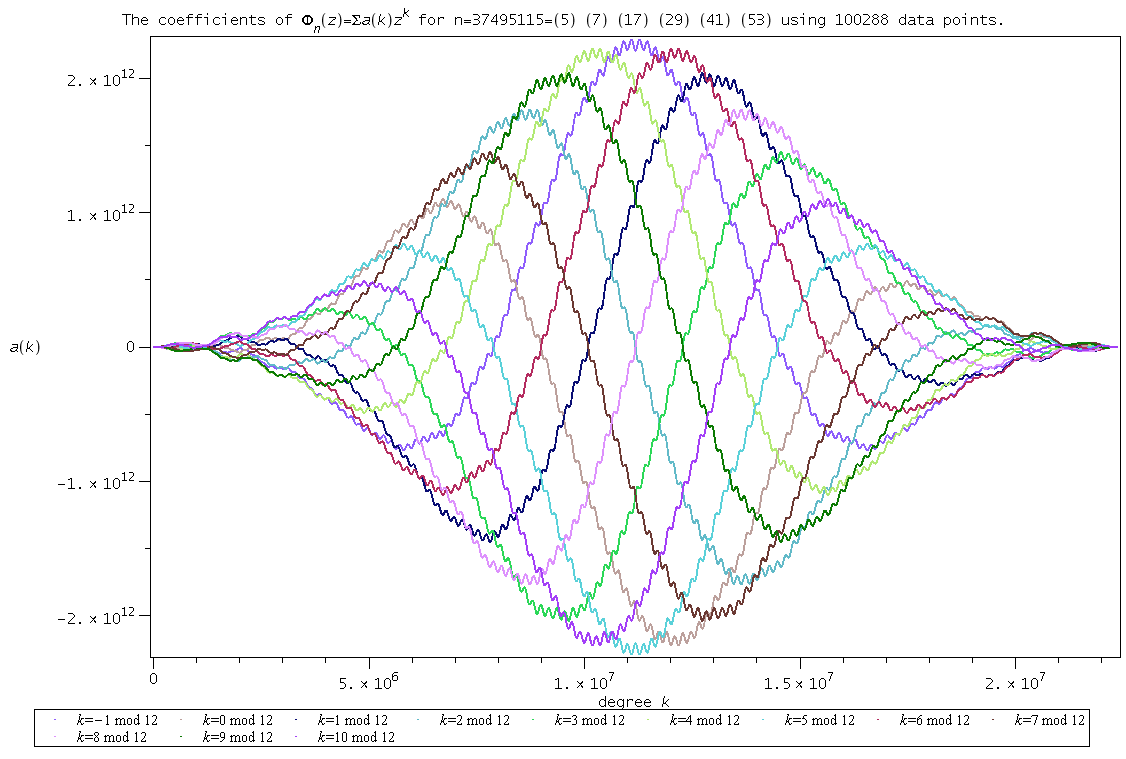
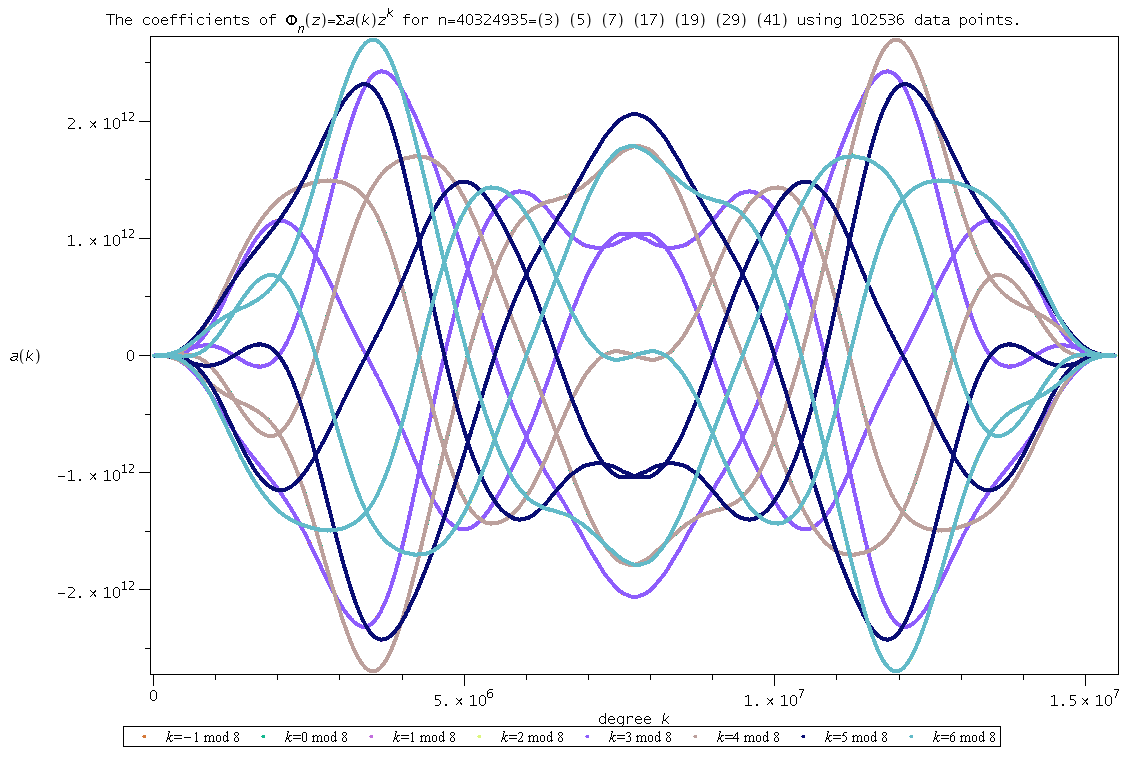
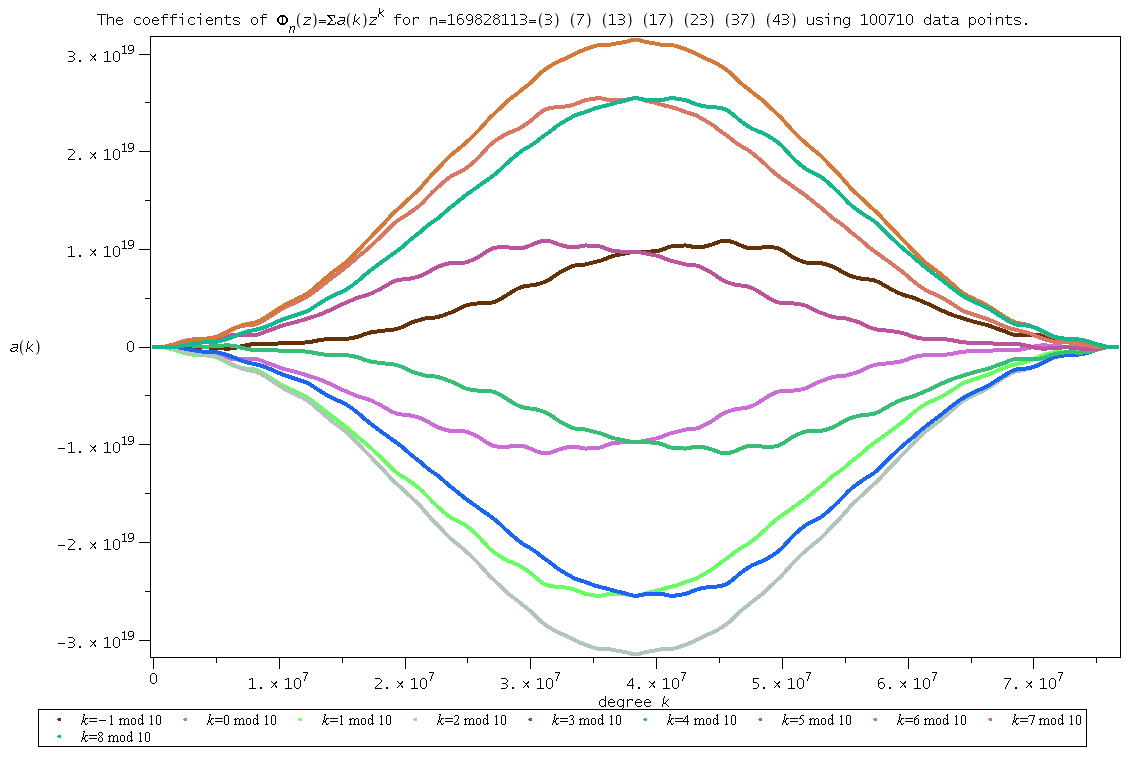
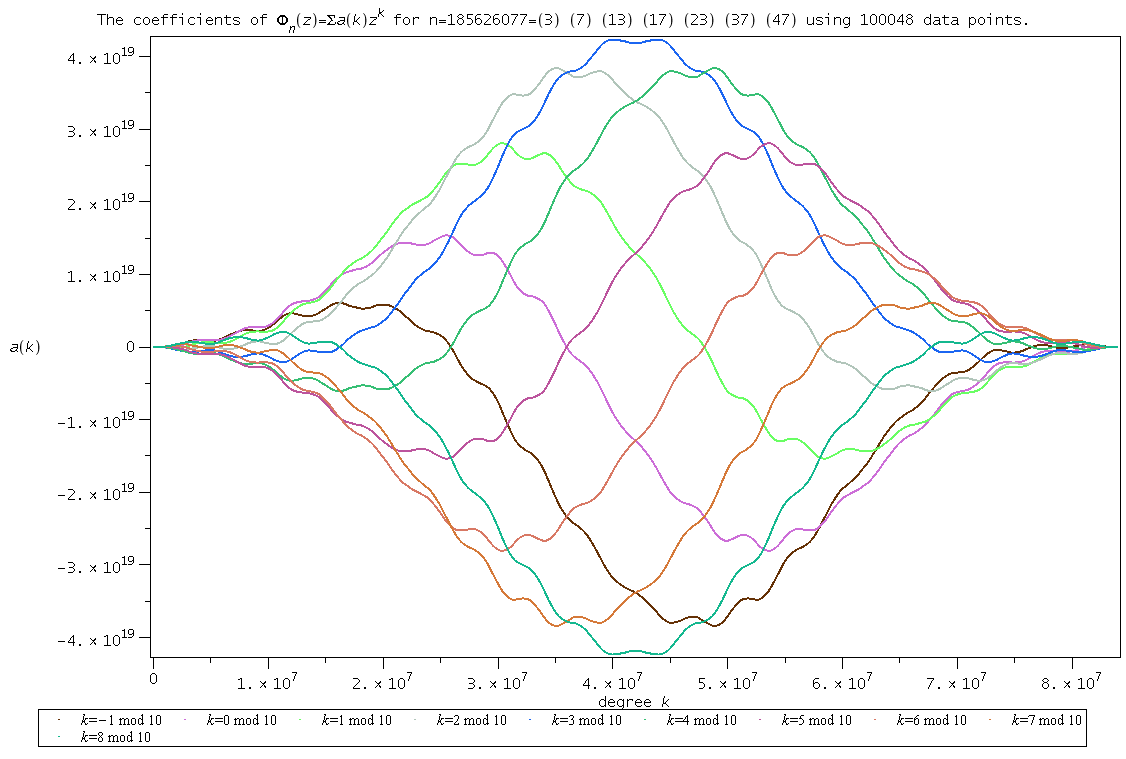
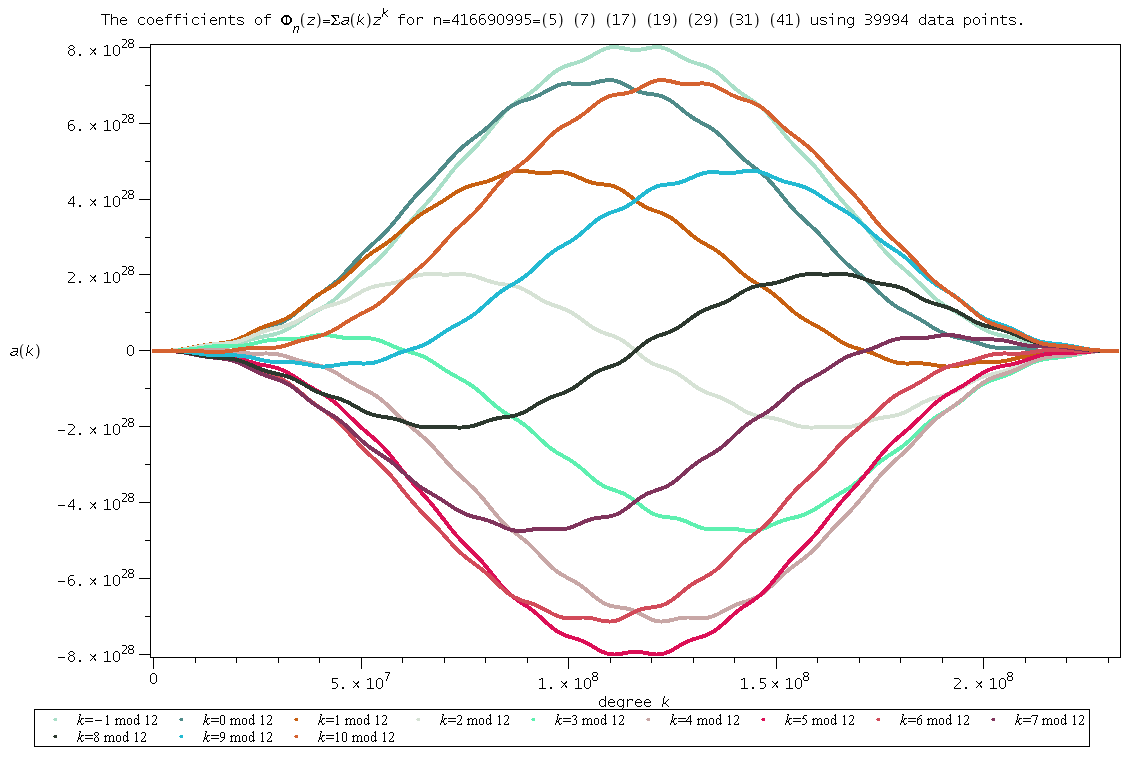
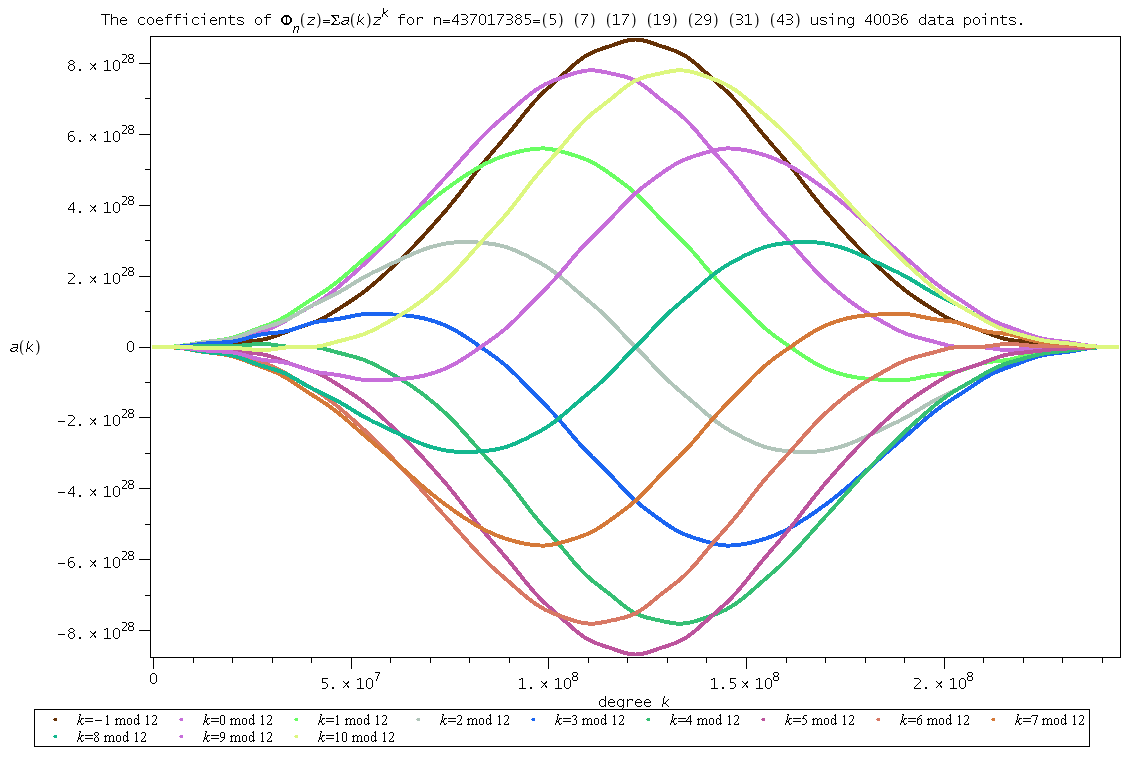
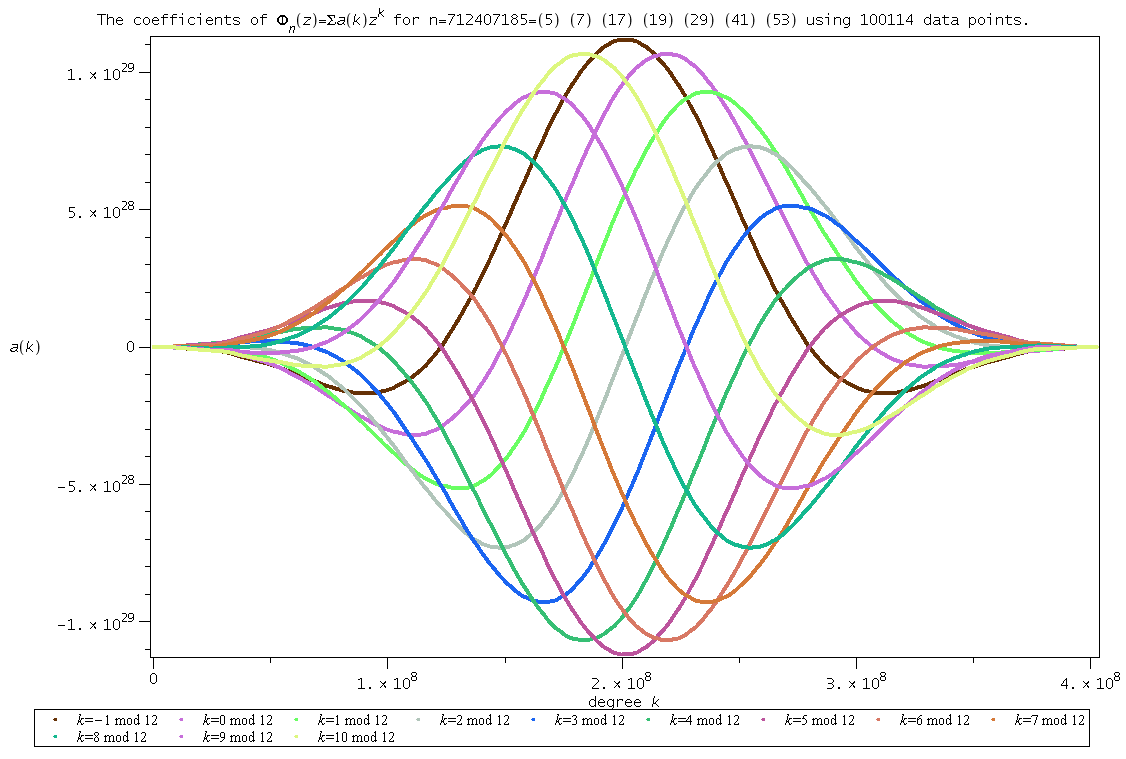
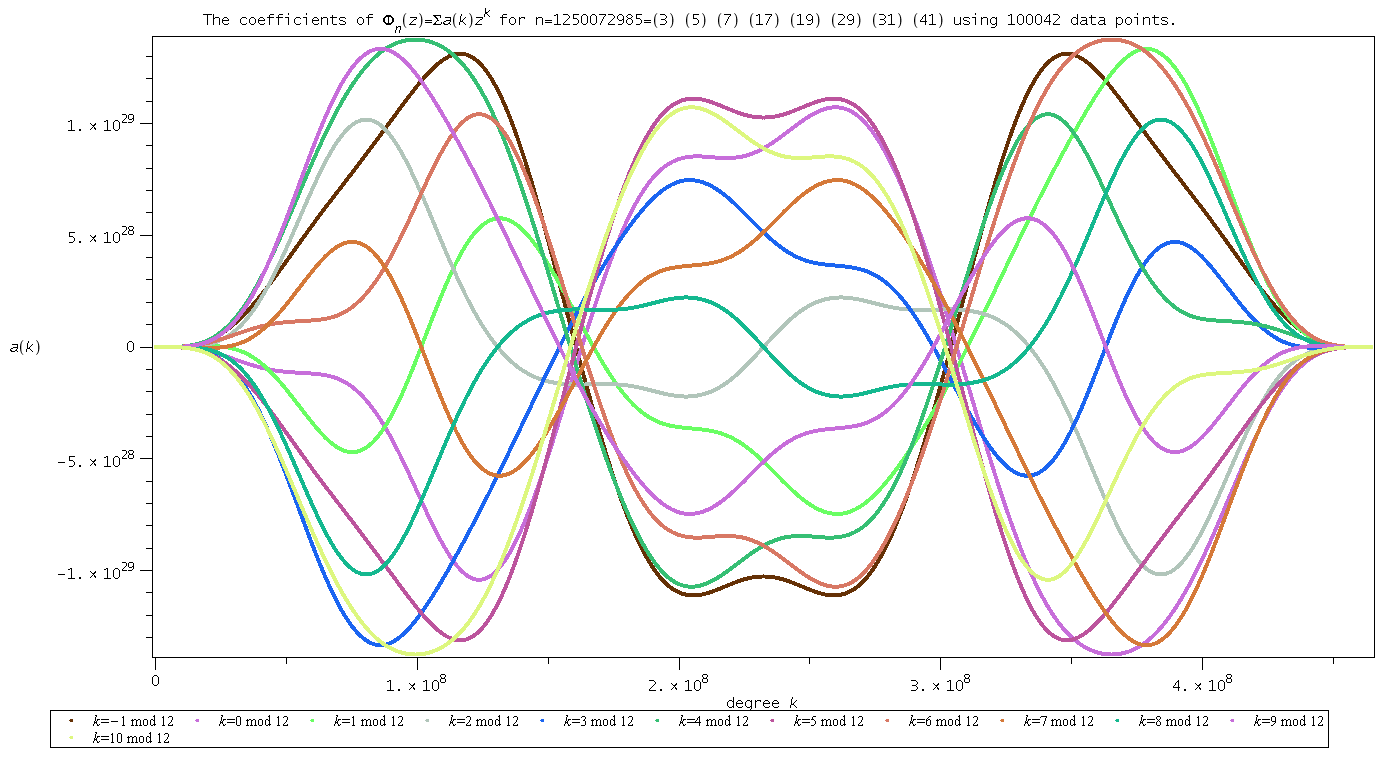
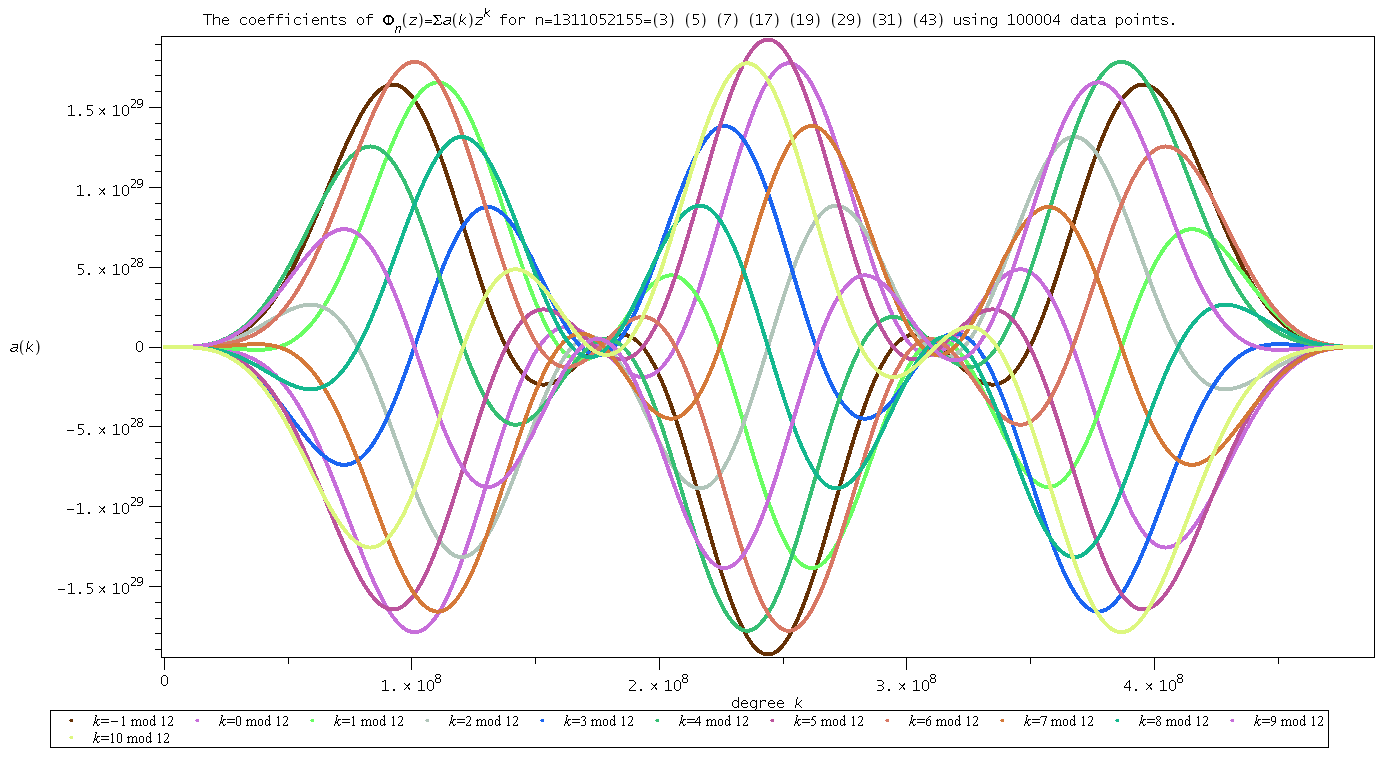
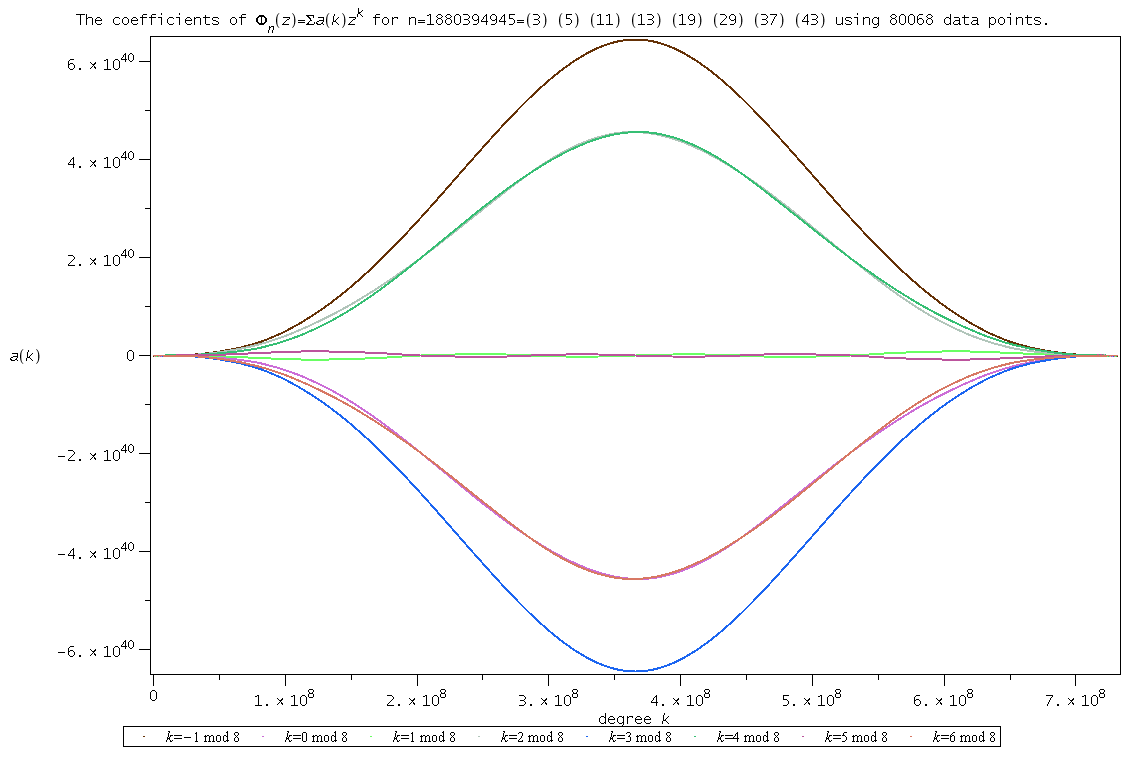
Here are the plots of $\Phi_n(z)$, for $n$ a product of the first $k$ primes, for $k$ from 4 to 9:

---