![[Shownotes]](../gif/annotate/shide-71.gif)
 are thus aperiodic. We
examine some beautiful examples, beginning with one due to Euler:
are thus aperiodic. We
examine some beautiful examples, beginning with one due to Euler:
 .
The elements of the orbit of this initial point are always of the form
.
The elements of the orbit of this initial point are always of the form
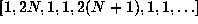 ,
, 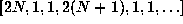 ,
or
,
or 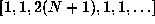 ,
which tend to 1, 0, and
,
which tend to 1, 0, and 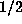 , respectively. Thus the
, respectively. Thus the
 -limit set
of this orbit is the set
-limit set
of this orbit is the set 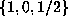 , which, unlike the
, which, unlike the  -
limit sets of continuous maps, is not invariant
under the Gauss
map since
-
limit sets of continuous maps, is not invariant
under the Gauss
map since  so G applied to this set simply gives
0. In other words, we have an
asymptotically periodic orbit which is
not asymptotic to a real orbit of the map. This cannot happen for a
discrete dynamical system with a continuous map.
so G applied to this set simply gives
0. In other words, we have an
asymptotically periodic orbit which is
not asymptotic to a real orbit of the map. This cannot happen for a
discrete dynamical system with a continuous map.
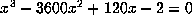 , then
, then

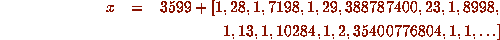 which has very large entries placed irregularly throughout. This
intermittency is a typical feature of a chaotic system [10].
which has very large entries placed irregularly throughout. This
intermittency is a typical feature of a chaotic system [10].
 is not known, in the sense that no pattern has
been identified. It begins
is not known, in the sense that no pattern has
been identified. It begins 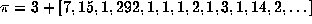 and some 17,000,000 elements of this continued fraction have been computed
by Gosper (see [4]). There are many open questions
about this continued fraction---for example, it is not known if the
elements of the continued fraction are bounded.
and some 17,000,000 elements of this continued fraction have been computed
by Gosper (see [4]). There are many open questions
about this continued fraction---for example, it is not known if the
elements of the continued fraction are bounded.